Heritability as a Phenotype x Genotype correlation
Consider
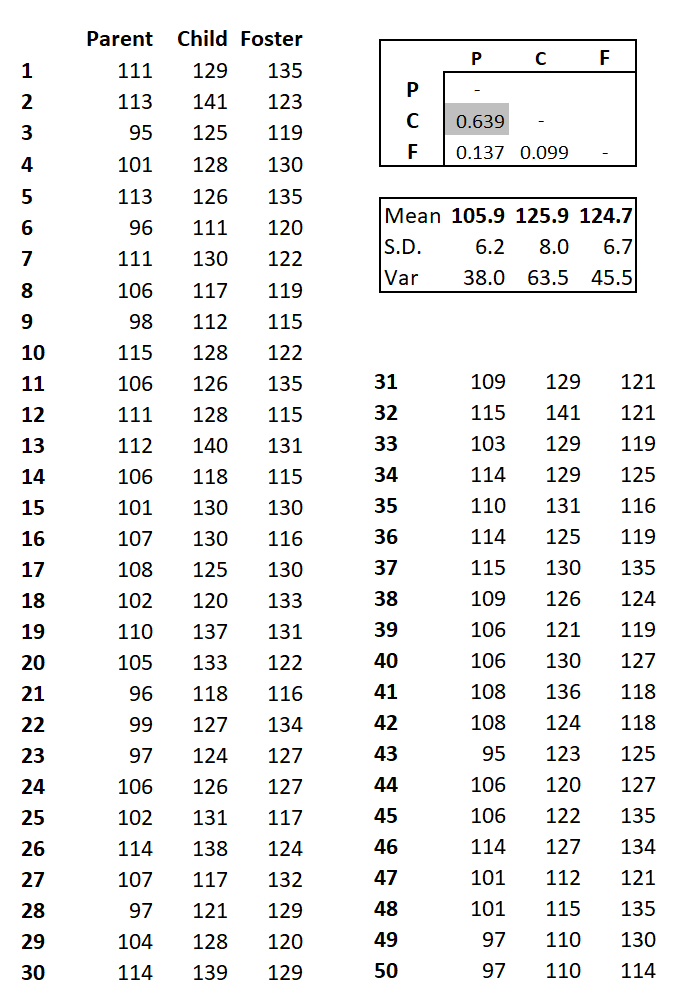
the educational test
scores ('IQs') for 50 children born to one set of
parents and fostered by another set. The mean score of the
birth parents (P)
is 105. The mean
score of the foster parents (F) is 125, 20 points higher
than that of the birth parents. After adoption, the
children (C) are provided with an "enriched"
environment. Upon testing, they collectively achieve the
same average score (125)
as their adoptive parents,again 20 points higher than
their birth parents.
What does this tell us about the heritability of test scores? Despite the mean difference of 20 points, the correlation r (which is equivalent to heritability, h2) between birth parents and their children is rPxC = 0.639. The adopted children collectively achieve an identical mean score as the foster parents, however the correlation between them is a negligible rCxF = 0.099.
That is, a trait that is perfectly heritable may also be perfectly changeable by modification of the environment. Enrichment per se improves mean test scores for the group, but is not predictable for any particular child.
It might be that adoptive children are (consciously or unconsciously) assigned foster parents from birth parents with similar socio-economic backgrounds as their birth parents. This might result in a similarity in their mean test scores. However, the correlation is also a negligible rpxf = 0.137.
HOMEWORK: The calculation of heritability as a correlation is straightforward as a spreadsheet problem, and is instructive from statistical first principles.
What does this tell us about the heritability of test scores? Despite the mean difference of 20 points, the correlation r (which is equivalent to heritability, h2) between birth parents and their children is rPxC = 0.639. The adopted children collectively achieve an identical mean score as the foster parents, however the correlation between them is a negligible rCxF = 0.099.
That is, a trait that is perfectly heritable may also be perfectly changeable by modification of the environment. Enrichment per se improves mean test scores for the group, but is not predictable for any particular child.
It might be that adoptive children are (consciously or unconsciously) assigned foster parents from birth parents with similar socio-economic backgrounds as their birth parents. This might result in a similarity in their mean test scores. However, the correlation is also a negligible rpxf = 0.137.
HOMEWORK: The calculation of heritability as a correlation is straightforward as a spreadsheet problem, and is instructive from statistical first principles.
Numerical
example after Griffiths et al. (2002); All text material
©2024 by Steven M. Carr